Intermediate Value Theorem (IVT):
Let f be continuous on a closed interval [a, b]. Pick a y-value M, somewhere between f(a) and f(b). The Intermediate Value Theorem says there has to be some x-value, c, with a < c < b and f(c) = M. We'll use "IVT" interchangeably with Intermediate Value Theorem. It's just much easier to use an abbreviation.
Here's what's going on, in pictures. Start with a continuous function f on a closed interval [a, b]:
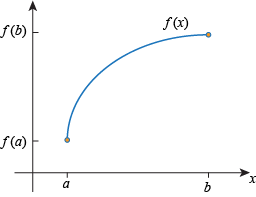
Mark on the y-axis where f(a) and f(b) are:
Pick any value of M strictly in between f(a) and f(b):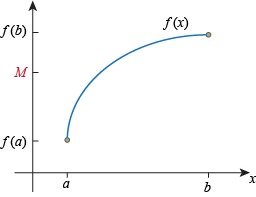
Draw a horizontal dashed line at height y = M. The IVT guarantees that this dashed line will hit the graph of f. In other words, the IVT guarantees the existence of some value c strictly in between a and b where the function value is M:

We've said a continuous function is one we can draw without lifting our pen from the paper. The IVT states this more precisely. If a continuous function starts at f(a) and ends at at f(b), then as x travels from a to b the function must hit every y value in between f(a) and f(b):

If a function on [a, b] skips a value, that function must be discontinuous:
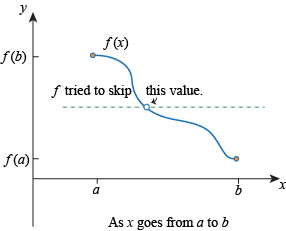
The statement of the theorem may be a little confusing, but with a few pictures this theorem shouldn't be much of a surprise. We're just making the whole notion of "drawing a continuous function without lifting our pencils" a little more formal.











